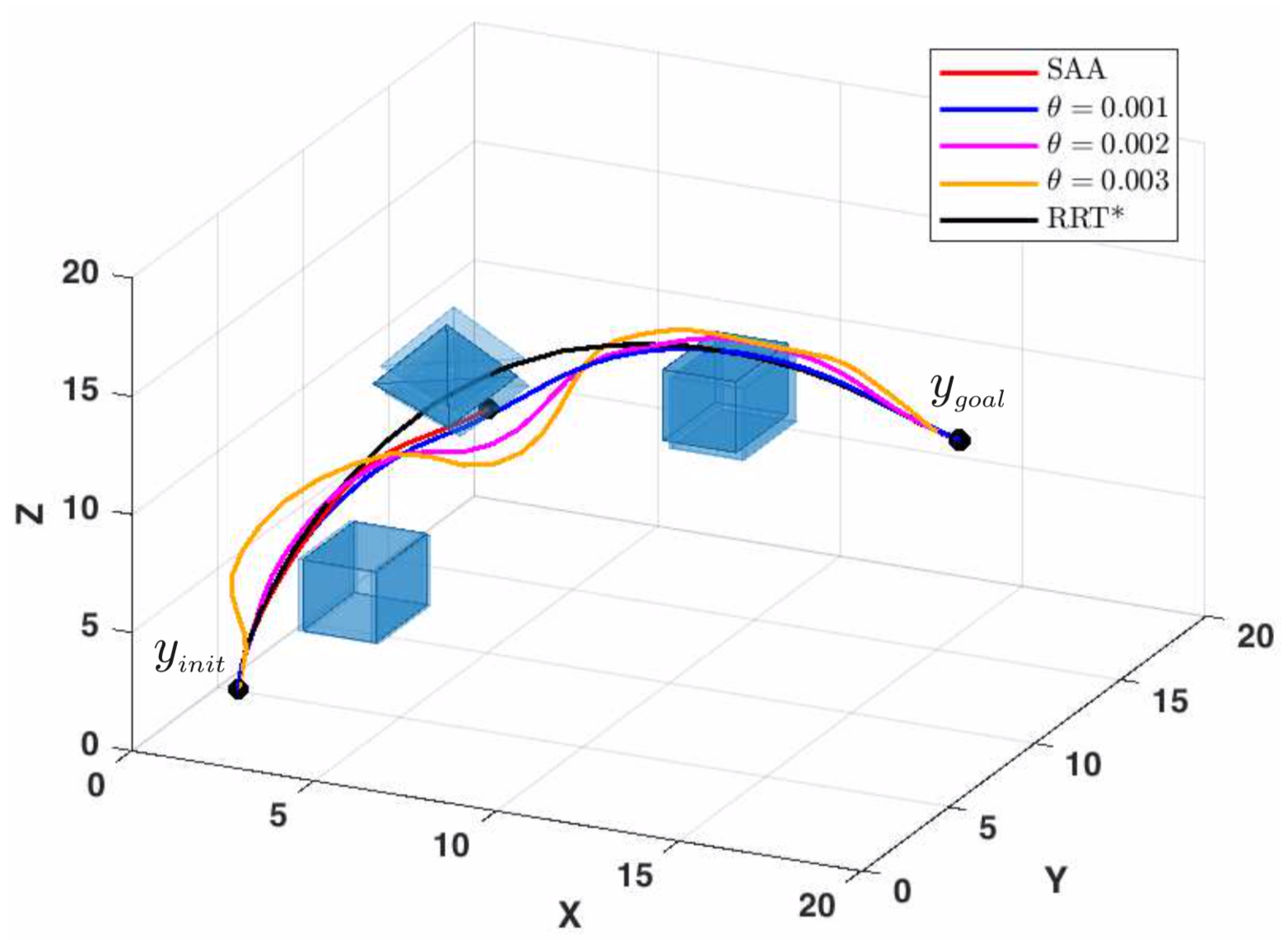
The paper “Wasserstein distributionally robust motion planning and control with safety constraints using conditional value-at-risk”, authored by Astghik Hakobyan, and Insoon Yang, has been accepted to the IEEE International Conference on Robotics and Automation (ICRA). Even when the sample size is very small (e.g., 5 data points), the proposed method can successfully avoid randomly moving obstacles with a guarantee of out-of-sample risk, while its sample average approximation counterpart fails to do so.

Abstract: In this paper, we propose an optimization-based decision-making tool for safe motion planning and control in an environment with randomly moving obstacles. The unique feature of the proposed method is that it limits the risk of unsafety by a pre-specified threshold even when the true probability distribution of the obstacles’ movements deviates, within a Wasserstein ball, from an available empirical distribution. Another advantage is that it provides a probabilistic out-of-sample performance guarantee of the risk constraint. To develop a computationally tractable method for solving the distributionally robust model predictive control problem, we propose a set of reformulation procedures using (i) the Kantorovich duality principle, (ii) the extremal representation of conditional value-at-risk, and (iii) a geometric expression of the distance to the union of halfspaces. The performance and utility of this distributionally robust method are demonstrated through simulations using a 12D quadrotor model in a 3D environment.